The first thing we did as we designed Chocolate Chip Cookie Factory: Place Value was envision how we would use the game ourselves as educators. While Chocolate Chip Cookie Factory: Place Value can be used as a fun game to engage kids and get them to practice their math facts, it can also help kids explore, develop, and apply place value concepts… building a foundation for adding, subtracting, multiplying, and dividing multi-digit numbers later in the classroom.

While hands-on discovery is crucial in the learning process, so is building and testing theories and practicing other metacognitive skills. Therefore, we created a practice mode within the game where an adult and a child (or two children on their own) can sit down to share, discuss, and experiment with new strategies together. This practice mode simply turns off the game’s clock and gives the player unlimited time to solve the problems and clear a round.

Next, we developed this brief learning guide. Parents are often uncertain about how much guidance to give a child during the discovery process. This learning guide lays out simple, scaffolded learning progressions that parents and teachers can use to guide a child toward conceptual understanding without telling the child what to do and depriving him or her of a rich learning experience.

If you are looking for a learning tool with more explicit instruction in place value and direct links to paper-and-pencil algorithms for multi-digit addition, subtraction, multiplication, and division, we recommend our interactive multitouch textbook: Place Value from the Ground Up. It covers all of the same skills and concepts addressed in this game.

Educational Theory
Learning Progressions
Extension Activities
Blackline Masters
Parallels to Paper-and-Pencil Algorithms
Common Core Standards

Educational Theory

Like other curricula from Vertical Learning Labs, the design of Chocolate Chip Cookie Factory: Place Value is based on the latest scientific research on how children learn. We believe that the theory of constructivism represents our best model of the learning process, and we use our understanding of that model to create learning environments that challenge and support kids to learn for themselves.

According to constructivism, learning is an active process. As we interact with the world, we build schemas (internal mental models or theories) that help us interpret what we experience. These schemas help us make sense of the world around us. Initially, a schema is very rudimentary. It may not work reliably. It may only work within very narrow contexts. Over time, as we apply our schema and test it against reality and in other contexts, we have the opportunity to revise and improve our schema: to make it more robust and more flexible. Good learners actively test their theories, looking for edge cases and points of cognitive dissonance, and revise their theories in the face of evidence.

If we think of constructing a skyscraper as an analogy for schema-building (the skyscraper represents the schema as a whole; each “floor” in the skyscraper represents an extension to the schema that makes it more powerful), then we can identify a number of instructional design decisions that we commonly make as teachers that can inhibit schema-building by our students. The first is trying to build a second floor before building the first. Either the second floor completely collapses or the student is forced to prop up sections of the second floor with whatever random stuff he can lay his hands on.

design error 1

The second is assuming that a floor is structurally sound before you have tried building on top of it. Evaluating the structural soundness of a floor by its external appearance is not a good idea. The only way to really evaluate the structural soundness of a floor is to start building on top of it. If things start to sag, then you will have to go back and re-engineer and rebuild parts of that floor.

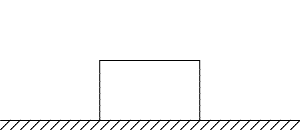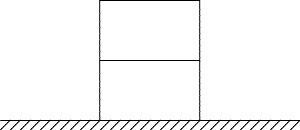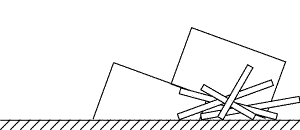
design error 2

The third is building each floor as a standalone structure instead of building floors on top of each other. This often happens when we don’t trust existing floors to support additional floors on top of them. This mean that the individual schemas never get very powerful, and the student never has a chance to test the structural soundness of his schemas and figure out how to re-engineer and rebuild them better.

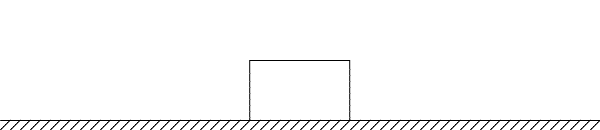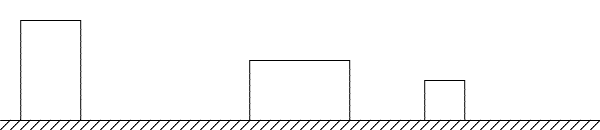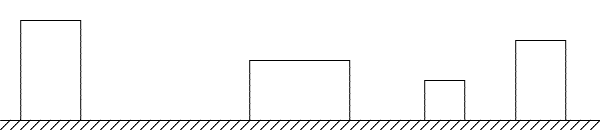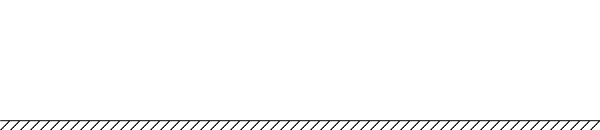
design error 3

The fourth is insisting that a foundation be solid enough to support ten floors before building the first floor on top of it. Okay, this is where our skyscraper construction analogy goes off the rails. If you were building a ten-story building, of course you would engineer and build the foundation so that it could support all ten floors.

Schema-building is a little different than that. When building a schema, you can’t identify the weak points in your schema until you build on top of it and see where it starts to fail. You have to build the first floor as best you can. Then test the first floor by starting to build the second floor on top of it. Depending on how the first floor holds up, you may have to go back and re-engineer and rebuild parts of the first floor so that it can support the additional weight of the second floor. Then, when the second floor is complete and the first floor has been rebuilt, you start adding the third floor and looking for weak points in the second floor and more weak points in the first floor. Schema-building is an iterative process.

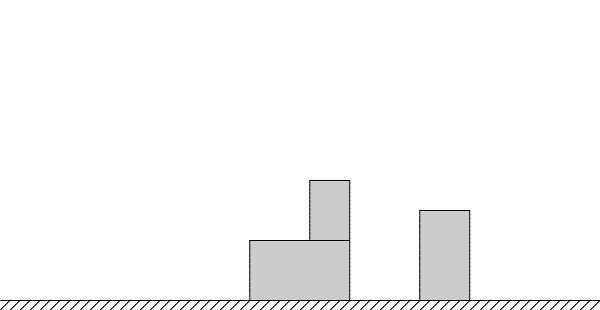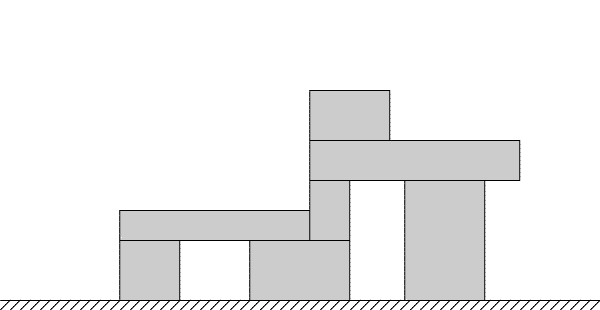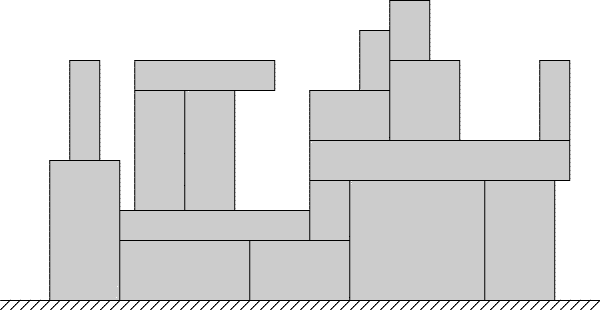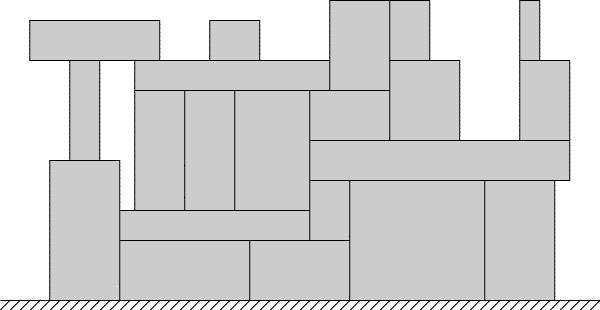
schema-building

Vertical learning is our approach to creating learning environments that encourage students to build and test their own schemas. We challenge students to build multi-story structures; and then we teach them how to go back and analyze what they’ve already built for weak points, and to re-engineer and rebuild as they go. And once they’ve built their first skyscraper, they always want to build more.

Learning Progressions

Developing an effective schema for working with multi-digit numbers is a long process with many steps. As an adult, it is often difficult to remember that process and to put ourselves in the shoes of a young, first-time learner. Chocolate Chip Cookie Factory: Place Value is designed to make place value concepts accessible without formal instruction. It builds on a kid’s own schema for working with concrete groups of objects. It separates the operation side of addition, subtraction, multiplication, and division from the accounting side. Most importantly, it enables kids to learn by making incremental revisions to their gaming strategies.

Counting

Stage 1: Count by ones

A student fills an order by counting and tapping out cookies one at a time. The student reads an order for 17 cookies as:

17 = cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie

Stage 2: Count by tens and hundreds

A student recognizes that 40 is “in the tens” and that 700 is “in the hundreds.” At this point, the student is probably just using pattern recognition and not necessarily reading the place values of the digits. The student knows that he can quickly reach 40 cookies by counting by tens and can reach 700 cookies by counting by hundreds.

40 = stack stack stack stack

700 = box box box box box box box

Stage 3: Use count by tens and hundreds to count faster

A student recognizes that 62 is “in the tens” and that 350 is “in the hundreds.” At this point, the student does not automatically recognize 62 as 6 tens + 2 ones or 350 as 3 hundreds + 5 tens (although he might know that if asked directly). However, he does know that counting to 62 by ones would take a long time. So he starts skip-counting by tens until he feels as though he is getting close to 62, and then switches over to counting by ones.

An aggressive student will occasionally skip-count too far, blowing past 62 to 70. A cautious student might stop skip-counting at 50 and then count by ones the rest of the way. If you notice this happening, you can slow a student down and get him thinking metacognitively by asking him to predict how many stacks of ten he will be able to add to an order before going over. When monitoring the count versus the order, ask the student: How do you know when you can add another stack and when another stack will put you over? Encourage him to test his hypothesis.

An order for 408 cookies would almost certainly confuse a student at this stage. Operating on cruise control, he would likely tap out 4 boxes of cookies and then automatically switch over to the stacks. Again, slow him down and get him thinking about what he is doing.

Stage 4: Parse numbers by reading digits and place value

After counting by tens and hundreds to count faster, a student will begin to notice that:

62 = 60 + 2 = stack stack stack stack stack stack cookie cookie

Actually, this is probably something that the student knew already (having learned it in class), but hasn’t begun applying in non-class contexts yet. The student will begin to read 62 as 60 + 2 and know that he can fill an order for 62 cookies by tapping out 6 stacks + 2 cookies.

Jumping to three-digit numbers, such as 384 may throw him off initially. Ask him to predict (hypothesize) what he could do to fill this order and then to test his hypothesis. He will see that:

384 = 300 + 80 + 4 = box box box stack stack stack stack stack stack stack stack cookie cookie cookie cookie

Moving to three-digit numbers will solidify his intuition that he can fill orders and interpret numbers just by reading the individual digits in a number. It will also get him in the habit of parsing or breaking numbers apart.

Stage 5: Generalize place value understanding

When a student parses a number like 384 and reads it as 300 + 80 + 4, it is still possible that he is using pattern recognition to know that the 3 is in the hundreds place, the 8 is in the tens place, and the 4 is in ones place. Students get drilled on two- and three-digit numbers in class, and our brains are hard-wired to recognize and apply these kinds of patterns.

To make sure that a student is applying place value understanding and not repeating a learned pattern, we ask him to fill orders for 38,426 cookies. While it is possible to memorize the place values of five-digit numbers on sight, most students will have to pick out the 3, ask themselves what its place value is, count over the number of digits, recognize that the 3 is in the ten thousands place, recognize that the 3 really has a place value of 30,000, and translate 30,000 into the cookie factory universe (3 crates).

To figure out the place value of the 8, the student could go through that whole process again. However, if he has truly understood and internalized place value, he should know that if the 3 is in the ten thousands place, then the 8 is in the thousands place and proceed from there. If you suspect that a student is identifying the place values for each digit in a number from scratch, ask him how he knows that the place value of the 3 is 30,000. Then ask him how he knows that the place value of the 8 is 8,000. If he explains that he found the position of the 8 by counting over the number of digits, ask him if he could have guessed the place value of the 8 once he knew that the place value of the 3 was 30,000.

Once a student has generalized his place value understanding, have fun with it. Extend the game into larger numbers. As we designed Chocolate Chip Cookie Factory: Place Value, we considered using planes to carry ten million cookies, cargo ships to carry a hundred million cookies, and rocket ships to carry a billion cookies. (What? The inhabitants of the Andromeda Galaxy need cookies too!) It builds confidence when a student realizes that he could fulfill a cookie order with a million digits in it if his cookie factory produced cookies in those quantities. An order with a million digits in it isn’t any harder to fill than an order with three digits in it; it just takes longer (and good organization). This confidence leads to automaticity, which reduces cognitive load and enables students to focus on problem solving.

Addition

Stage 1: Add by counting on

To fill an addition order for 16 + 35 cookies, a student adds 16 cookies to the order and then adds another 35 cookies to the order:

16 + 35 = stack cookie cookie cookie cookie cookie cookie stack stack stack cookie cookie cookie cookie cookie

You should notice that to fill an order for 16 + 35 cookies, the student doesn’t need to know the answer to 16 + 35. The student is simply applying his understanding of the addition operation. Counting the sum (the total number of cookies in the order) can come later.

Stage 2: Add by using addition facts and place value

To fill an addition order for 8 + 6 cookies, a student could add 8 cookies to the order and then add another 6 cookies to the order. This requires 14 taps. But if the student happens to know that 8 + 6 = 14 and that 14 = 10 + 4, then the student could fill the order in only 5 taps:

8 + 6 = 14 = 10 + 4 = stack cookie cookie cookie cookie

Wanting to get better at the game encourages the student to memorize and apply his addition facts, developing automaticity.

Stage 3: Add in other units

By translating numbers into the cookie factory universe, a student can figure out how to fill an order for 30 + 90 cookies. Since 30 cookies = 3 stacks and 90 cookies = 9 stacks, 30 + 90 = 3 stacks + 9 stacks = 12 stacks:

30 + 90 = stack stack stack stack stack stack stack stack stack stack stack stack

By working in the cookie factory universe, the student can see that adding 30 + 90 is the same as adding 3 + 9, just in different units or in a different place value.

If the student also happens to know that 3 + 9 = 12 and that 120 = 100 + 20, then the student could fill the order in only 3 taps:

30 + 90 = 120 = 100 + 20 = box stack stack

Stage 4: Add by combining hundreds with hundreds, tens with tens, and ones with ones

To fill an addition order for 16 + 35 cookies, a student can also add the stacks from the 16 and 35 orders first, and then add the cookies from the 16 and 35 orders second.

16 + 35 = 10 + 30 + 6 + 5 = stack stack stack stack cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie cookie

Adding all of the stacks and then all of the cookies at once, instead of switching back and forth, enables the student to fill the orders faster. (The number of taps is the same, but less movement is required.) It also helps him practice picking out digits by their position in a number.

And if he does know his addition facts, then the student could fill the order in only 6 taps instead of 15 taps:

16 + 35 = (10 + 30) + (6 + 5) = 40 + 11 = 40 + 10 + 1 = stack stack stack stack stack cookie

You should notice that the student does not have to hold all of the digits in his head. He can fill this order by first focusing on 10 + 30 = 40, and then by focusing on 6 + 5 = 11 = 10 + 1. Learning how to isolate and focus in on specific parts of the problem instead of trying to hold the entire problem in your head reduces cognitive load and helps you focus on problem solving.

Stage 5: Generalize addition by combining digits in the same place

To fill an addition order for 253,892 + 76,441 cookies, a student can generalize the process he developed in stage 4. We make this easier in levels 5 and 6 by lining up the digits for him. First, translate the order into the cookie universe:

253,892 + 76,441 = (2 pallets) + (5 crates + 7 crates) + (3 cartons + 6 cartons) + (8 boxes + 4 boxes) + (9 stacks + 4 stacks) +
(2 cookies + 1 cookie)

Do the mental math:

253,892 + 76,441 = (2 pallets) + (12 crates) + (9 cartons) + (12 boxes) + (13 stacks) + (3 cookies)

Apply place value to break up multi-digit numbers along the way:

253,892 + 76,441 = (2 pallets) + (1 pallet + 2 crates) + (9 cartons) + (1 carton + 2 boxes) + (1 box + 3 stacks) + (3 cookies)

Remember, the student can focus on the digits in one place at a time. He doesn’t have to hold the entire problem in his head, and he can go from left to right, from right to left, or choose the digits in any order he likes.

Students can practice this process using the extension activity, Column Addition and Multiplication in Two Steps (see below). This activity will help the student visualize the process using paper and pencil, and enable the student to find the actual sum.

Stage 6: Add by going right to left and carrying

Filling addition orders by adding digits separately can really speed things up, but sometimes you can go even faster.

58 + 45 = (50 + 40) + (8 + 5) = stack stack stack stack stack stack stack stack stack stack cookie cookie cookie

Notice that, to fill this order in stage 5, a student taps in 10 stacks + 3 cookies. But 10 stacks = 100 cookies = 1 box. The student could have saved himself a lot of time by tapping in 1 box + 3 cookies:

58 + 45 = box cookie cookie cookie = 103

To minimize the number of taps to enter an addition order, the student can start by adding the digits in the ones place (the cookies):

8 + 5 = 13

He taps in the three cookies, but doesn’t tap in the stack yet. He is going to “carry” the stack and add it to the other stacks in the order:

58 + 45 = (8 + 5) + (50 + 40) = 13 + (50 + 40) = 3 + (10 + 50 + 40)

Notice how the one stack from the 13 gets added to the five stacks from the 58 and the four stacks from the 45. 1 stack + 5 stacks + 4 stacks = 10 stacks = 1 box. Carrying is sometimes called regrouping or composing. The student is regrouping or composing 10 stacks into 1 box. So:

58 + 45 = cookie cookie cookie box

Filling addition orders this way means fewer taps, but it also means holding more numbers in your head. Reaching this stage by playing the Chocolate Chip Cookie Factory: Place Value is not very intuitive. Making the jump to stage 6 is much easier using the extension activity, Column Addition and Multiplication with Carrying (see below).

Multiplication

Stage 1: Multiply using repeated addition

Multiplying and adding muti-digit numbers share many of the stages. This is because one way to think of multiplication is as repeated addition. A student doesn’t have to have all of his multiplication facts memorized to fill a multiplication order. If he receives an order for 28 × 3 cookies, the student can simply tap in an order for 28 cookies three times:

28 × 3 = stack stack cookie cookie cookie cookie cookie cookie cookie cookie stack stack cookie cookie cookie cookie cookie cookie cookie cookie stack stack cookie cookie cookie cookie cookie cookie cookie cookie

Stage 2: Multiply by using multiplication facts and place value

But if a student happens to know that 2 stacks × 3 = 6 stacks and 8 cookies × 3 = 24 cookies, then he can save himself some time by tapping out 6 stacks + 2 stacks + 4 cookies:

28 × 3 = 60 + 24 = stack stack stack stack stack stack stack stack cookie cookie cookie cookie

By using place value and multiplication facts, the student went from 30 taps to down to 12 taps. And in the cookie business, time is money!

Stage 3: Multiply in other units

By translating numbers into the cookie factory universe, a student can figure out how to fill an order for 70 × 4 cookies. Since 70 cookies = 7 stacks, 70 × 4 = 7 stacks × 4 = 28 stacks = 2 boxes + 8 stacks:

70 × 4 = 280 = box box stack stack stack stack stack stack stack stack

By working in the cookie factory universe, the student can see that multiplying 70 × 4 is the same as multiplying 7 × 4, just in different units or in a different place value.

Stage 4: Generalize multiplication by multiplying each digit separately

To fill a multiplication order for 37,581 × 6 cookies, a student can generalize the process he developed in stage 2. First, translate the order into the cookie universe:

37,581 × 6 = (3 crates + 7 cartons + 5 boxes + 8 stacks + 1 cookie) × 6

Multiply each digit separately:

37,581 × 6 = (18 crates) + (42 cartons) + (30 boxes) + (48 stacks) + (6 cookies)

Apply place value to break up multi-digit numbers along the way:

37,581 × 6 = (1 pallet + 8 crates) + (4 crates + 2 cartons) + (3 cartons + 0 boxes) + (4 boxes + 8 stacks) + (6 cookies)

Stage 5: Multiply going right to left and carrying

Filling multiplication orders by multiplying digits separately can really speed things up, but sometimes you can go even faster.

34 × 3 = (30 × 3) + (4 × 3) = stack stack stack stack stack stack stack stack stack stack cookie cookie

Notice that, to fill this order in stage 4, a student taps in 10 stacks + 2 cookies. But 10 stacks = 100 cookies = 1 box. The student could have saved himself a lot of time by tapping in 1 box + 2 cookies:

34 × 3 = box cookie cookie = 102

To minimize the number of taps to enter a multiplication order, the student can start by multiplying the number of cookies:

4 × 3 = 12

He taps in the two cookies, but doesn’t tap in the stack yet. He is going to “carry” the stack and add it to the other stacks in the order:

34 × 3 = (4 × 3) + (30 × 3) = 12 + (30 × 3) = 2 + (10 + 90)

Notice how the one stack from the 12 gets added to the nine stacks from the 3 stacks multiplied by 3. 1 stack + 9 stacks = 10 stacks = 1 box. After carrying:

34 × 3 = cookie cookie box

Filling multiplication orders this way means fewer taps, but it also means holding more numbers in your head. Reaching this stage by playing the Chocolate Chip Cookie Factory: Place Value is not very intuitive. Making the jump to stage 5 is much easier using the extension activity, Column Addition and Multiplication with Carrying (see below).

Subtraction

Stage 1: Subtract by opening boxes and stacks

Delivering subtraction orders is fairly straightforward when a student is operating in the cookie factory universe. Delivering an order is the same as counting out an order. The only real difference is that sometimes the student needs to open up a box of cookies to get more stacks or open up a stack of cookies to get more cookies. This should be very intuitive as it builds on top of the student’s own schema for working with real-world cookie boxes. For example, to deliver an order for 32 cookies to this house:

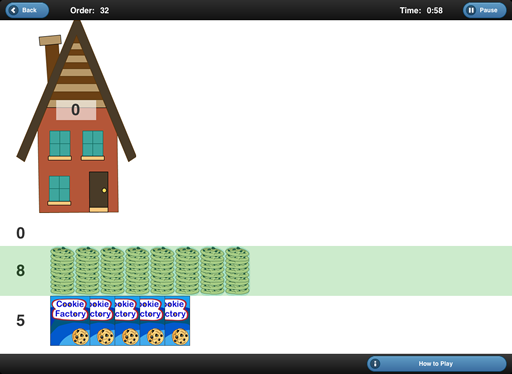
The student can deliver 30 cookies by selecting the stacks and tapping on the house three times:

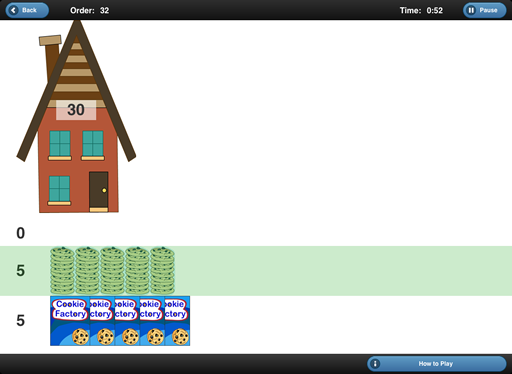
To deliver the two remaining cookies, the student needs two more cookies. To get them, he can double tap on a stack to open it:

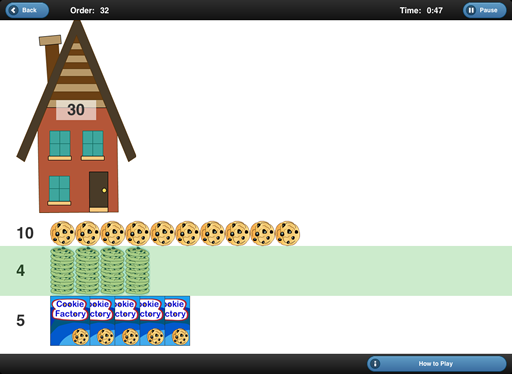
Now he can finish delivering the order.

Stage 2: Generalize subtraction by subtracting digits and borrowing when necessary

Delivering an order for 23,894 cookies when you have 62,500 cookies on board your truck is the same as subtracting 62,500 − 23,894. Delivering the subtraction order seems easier only because no one is asking you to count how many cookies you still have on board the truck after the delivery has been made. Students can practice finding the difference of a subtraction problem using the extension activity, Column Subtraction (see below).

Even though it feels easier, a student is still performing the exact same steps filling the delivery order as he would be doing solving the subtraction problem with pencil and paper. As the delivery orders get larger, the student will begin generalizing the process he developed in stage 1.

First, he can deliver 4 cookies. To do this, the student needs to open a box of cookies to get 10 stacks, and then open one of those stacks to get 10 cookies. Opening a box or stack is also known as borrowing, regrouping, or decomposing.

62,500 − 4 = (6 crates + 2 cartons + 5 boxes + 0 stacks + 0 cookies) − 4 cookies
= (6 crates + 2 cartons + 4 boxes + 10 stacks + 0 cookies) − 4 cookies
= (6 crates + 2 cartons + 4 boxes + 9 stacks + 10 cookies) − 4 cookies
= (6 crates + 2 cartons + 4 boxes + 9 stacks + 6 cookies)

Then, he can deliver 9 stacks.

62,496 − 90 = (6 crates + 2 cartons + 4 boxes + 9 stacks + 6 cookies) − 9 stacks
= (6 crates + 2 cartons + 4 boxes + 0 stacks + 6 cookies)

Then, he can deliver 8 boxes. To do this, the student needs to open a carton to get 10 more boxes.

62,406 − 800 = (6 crates + 2 cartons + 4 boxes + 0 stacks + 6 cookies) − 8 boxes
= (6 crates + 1 carton + 14 boxes + 0 stacks + 6 cookies) − 8 boxes
= (6 crates + 1 carton + 6 boxes + 0 stacks + 6 cookies)

Then, he can deliver 3 cartons. To do this, the student needs to open a crate to get 10 more cartons.

61,606 − 3,000 = (6 crates + 1 carton + 6 boxes + 0 stacks + 6 cookies) − 3 cartons
= (5 crates + 11 cartons + 6 boxes + 0 stacks + 6 cookies) − 3 cartons
= (5 crates + 8 cartons + 6 boxes + 0 stacks + 6 cookies)

Finally, he can deliver 2 crates.

58,606 − 20,000 = (5 crates + 8 cartons + 6 boxes + 0 stacks + 6 cookies) − 2 crates
= (3 crates + 8 cartons + 6 boxes + 0 stacks + 6 cookies)

The student does not have to subtract from right to left. However, he will find that it is more efficient. The key thing is that the student develops the habit of borrowing when necessary by building on top of an existing, robust schema.

Division

Stage 1: Divide by distributing cookies, stacks, and boxes

A student doesn’t have to have all of his division facts memorized to deliver a division order. If he receives an order for 6 ÷ 2 cookies, the student can simply start by delivering one cookie to each house:

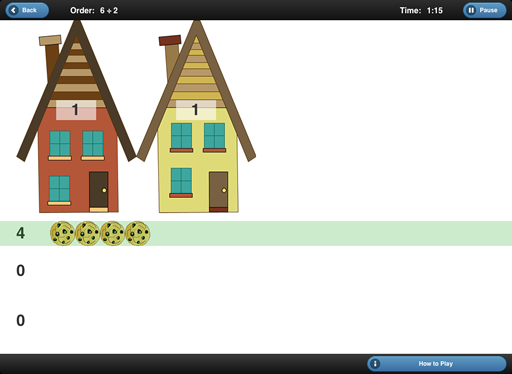
Then he can deliver a second cookie to each house:

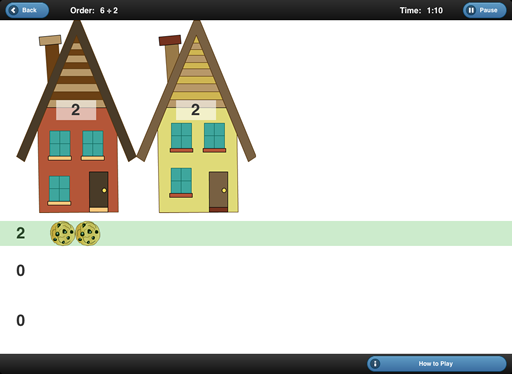
And he keeps going until he doesn’t have any more cookies left. In this case, each house will get 3 cookies.

Stage 2: Divide leftover stacks and boxes by opening them up

A student can divide 96 cookies equally between four houses by dividing the 9 stacks first:

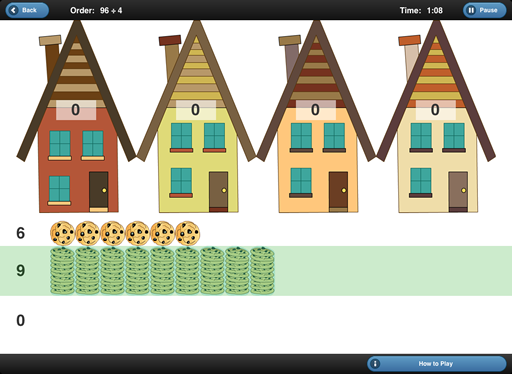
The student can give 2 stacks to each house. That leaves 1 stack and 6 cookies leftover:

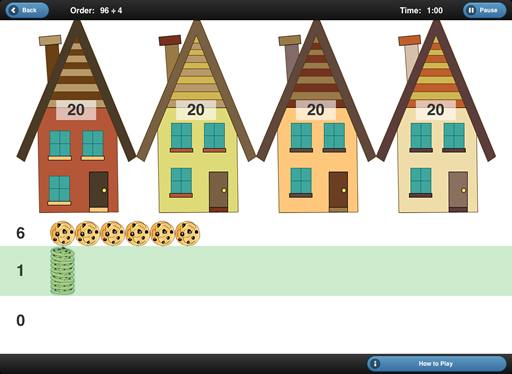
In order to divide the cookies in the leftover stack, the student needs to open it and combine those 10 cookies with the other cookies. Again, the student is applying his schema for working with cookie stacks in the real-world. He now has 16 cookies to divide:

Each house gets 24 cookies (2 stacks + 4 cookies), and there are no leftovers.

Stage 3: Generalize division by dividing digits from left to right and opening leftovers

To deliver a shipment of 37,302 cookies to 3 houses, a student can generalize the process he developed in stage 2. Initially, the student may not think to go from left to right (instead, dividing from right to left or dividing whatever he can before opening anything), but he will quickly discover that it is far more efficient to divide the largest units of cookies first.

First, he can deliver 1 crate to each house. This leaves him with 7 cartons + 3 boxes + 0 stacks + 2 cookies to deliver.

37,302 ÷ 3 = (3 crates + 7 cartons + 3 boxes + 0 stacks + 2 cookies) ÷ 3 = 1 crate + R(7 cartons + 3 boxes + 0 stacks + 2 cookies)

Then, he can deliver 2 cartons to each house. This leaves him with 1 carton + 3 boxes + 0 stacks + 2 cookies to deliver.

(7 cartons + 3 boxes + 0 stacks + 2 cookies) ÷ 3 = 2 cartons + R(1 carton + 3 boxes + 0 stacks + 2 cookies)

Then, he can open the leftover carton and combine those 10 boxes with the other 3 boxes.

(1 carton + 3 boxes + 0 stacks + 2 cookies) = (13 boxes + 0 stacks + 2 cookies)

Then, he can deliver 4 boxes to each house. This leaves him with 1 box + 0 stacks + 2 cookies to deliver.

(13 boxes + 0 stacks + 2 cookies) ÷ 3 = 4 boxes + R(1 box + 0 stacks + 2 cookies)

Then, he can open the leftover box.

(1 box + 0 stacks + 2 cookies) = (10 stacks + 2 cookies)

Then, he can deliver 3 stacks to each house. This leaves him with 1 stack + 2 cookies to deliver.

(10 stacks + 2 cookies) ÷ 3 = 3 stacks + R(1 stack + 2 cookies)

Then, he can open the leftover stack and combine those 10 cookies with the other 2 cookies.

(1 stack + 2 cookies) = (12 cookies)

Finally, the student can deliver 4 cookies to each house without any leftovers.

(12 cookies) ÷ 3 = 4 stacks

In the end, the student has delivered 1 crate + 2 cartons + 4 boxes + 3 stacks + 4 cookies to each house. You should notice that the student doesn’t need to find the quotient to deliver a division order. Students can practice this process and finding the quotient using the extension activity, Column Division (see below).

Extension Activities

These are some activities that you can use to help a student summarize, generalize, and build on top of what he or she has learned while playing Chocolate Chip Cookie Factory: Place Value. Most of these activities connect to the activities a student will be doing in the classroom.

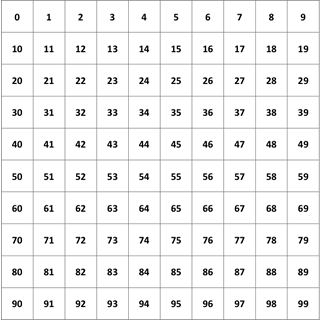
Hundreds Chart

A hundreds chart is a useful tool for students learning to count by ones and tens. The standard hundreds chart, found in many elementary math curricula, consists of the numbers 1-100. At Vertical Learning Labs, we prefer a chart that consists of the numbers 0-99. We feel that this chart is more extensible. However, if you are going to stick to ones and tens, it may be better to use the standard hundreds chart so that students don’t get confused by seeing two versions.

Students can use a hundreds chart to count forward (add) or count backward (subtract). To add 37 + 24, start at 37 and count forward by one 24 times.

37 + 24

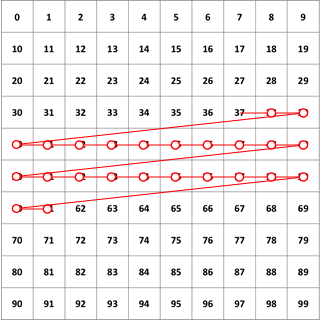
As a student uses the chart, he or she will discover that there is a short cut for counting by tens: moving down a row. Making this discovery will help a student see that you can add ten simply by incrementing the digit in the tens place and leaving the digit in the ones place alone. So to add 37 + 24, you could start at 37, count forward by one 4 times, and then count forward by ten 2 times; or you could start at 37, count forward by ten 2 times, and then count forward by one 4 times.

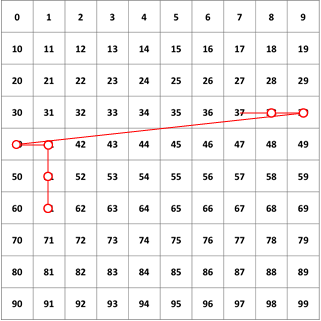
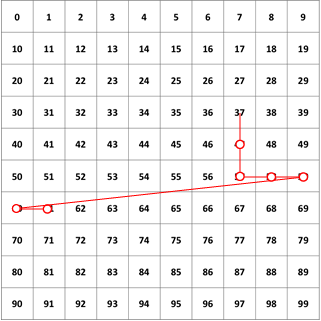
37 + 4 + 20 37 + 20 + 4

Subtraction works the same way, except you move backward when counting. The 0-999 booklet can be used if you want to extend this model for addition and subtraction to three-digit numbers. Count by hundreds by turning pages in the booklet.

Using Visual Representations

To help students stay immersed in the cookie factory universe while doing addition, subtraction, multiplication, and division problems outside of the game, print and cut out these handy drawings of cookies, stacks, and boxes… or have them draw their own. You could also use physical objects, such as base ten blocks, to represent cookies, stacks, and boxes. Get creative. Have students write and illustrate their own stories or word problems in the cookie factory universe.

Column Addition and Multiplication in Two Steps

Once students are ready to move to the next level of abstraction, they can represent addition, subtraction, multiplication, and division in columns. Recording problems in columns will help them keep track of and calculate sums, differences, products, and quotients.

We recommend starting out by separating addition and multiplication into two steps instead of carrying as you go. To add 357 + 286, represent the problem in cookies, stacks, and boxes. Then, combine the cookies with cookies, the stacks with stacks, and the boxes with boxes. Finally, regroup and count the total number of cookies you have.

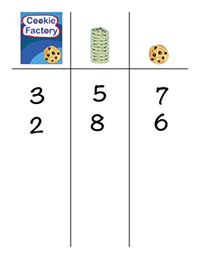
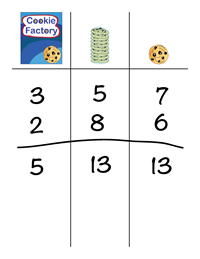
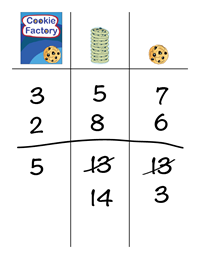
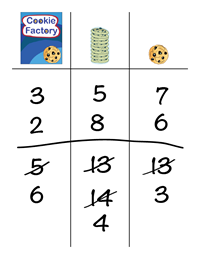
adding: set up adding: step 1 adding: step 2a adding: step 2b

The second step, regrouping, is not actually necessary. You can count by ones, tens, and hundreds to figure out how many cookies you have when you have 5 boxes + 13 stacks + 13 cookies, but students will discover that regrouping makes their lives is easier.

To multiply 254 × 3, you can either multiply each digit or use repeated addition. Then, regroup and count the total number of cookies you have.

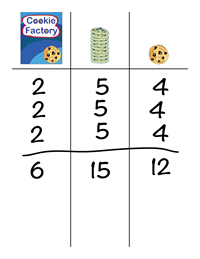
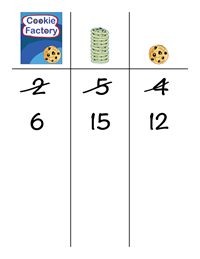
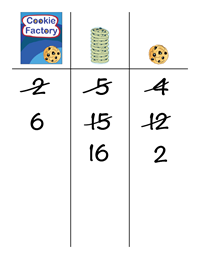
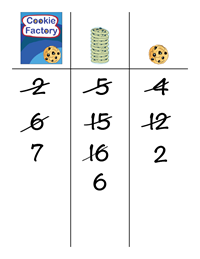
repeated addition multiplying: step 1 multiplying: step 2a multiplying: step 2b

To save paper, we use SmartPAL® Jr. sleeves from EAI Education at Vertical Learning Labs. You can find similar products at most educational supply stores.

Column Subtraction

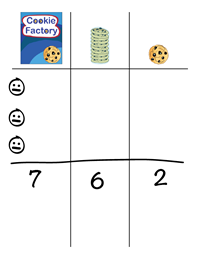
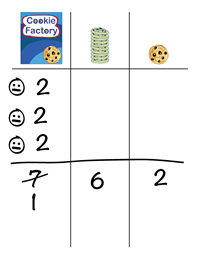
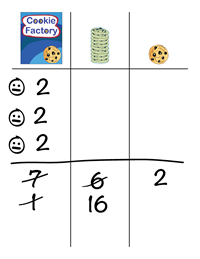
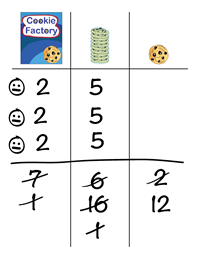
To subtract 357 − 286, represent the problem in cookies, stacks, and boxes. Then, subtract digits from right to left, borrowing when necessary. Finally, count how many cookies you have left.

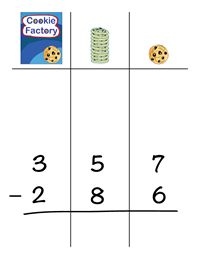
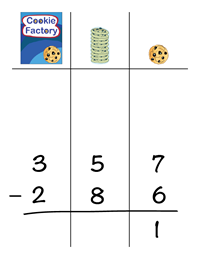
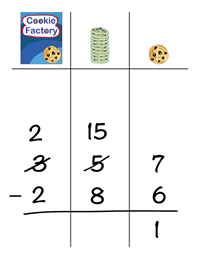
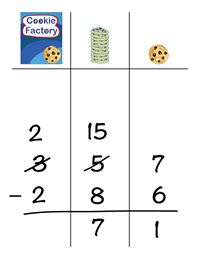
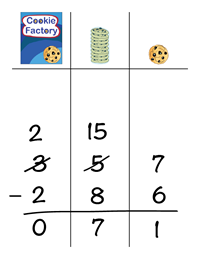
subtracting: set up subtracting: step 1a subtracting: step 1b subtracting: step 1c subtracting: step 1d

To save paper, we use SmartPAL® Jr. sleeves from EAI Education at Vertical Learning Labs. You can find similar products at most educational supply stores.

Column Division

To divide 762 ÷ 3, represent the problem in cookies, stacks, and boxes. Then, start by dividing the boxes and opening any leftover boxes, dividing the stacks and opening any leftover stacks, and dividing the cookies. Finally, count how many cookies each person received. Initially, we only give students division problems that divide evenly, but you can introduce the concept of remainders when you feel they are ready.

dividing: set up dividing: step 1a dividing: step 1b dividing: step 2a dividing: step 2b dividing: step 3a

To save paper, we use SmartPAL® Jr. sleeves from EAI Education at Vertical Learning Labs. You can find similar products at most educational supply stores.

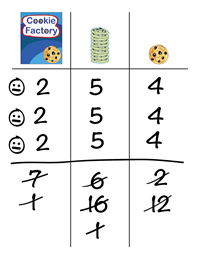
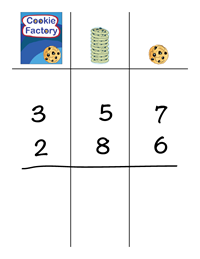
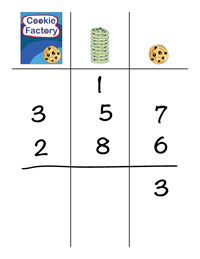
Column Addition and Multiplication with Carrying

Once students are comfortable adding and multiplying in two steps (doing their regrouping only after all of the addition or multiplication is done), you can show them how they can add and multiply in one step (saving time and paper) by carrying.

To add 357 + 286, represent the problem in cookies, stacks, and boxes. Then, combine the cookies with cookies, record the 3 cookies, and carry the 1 stack you can form by regrouping. Then, combine the stacks with stacks, record the 4 stacks, and carry the 1 box you can form by regrouping. Then, combine the boxes with boxes and record the 6 boxes. Finally, count the total number of cookies you have.

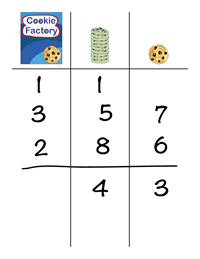
adding: set up adding: step 1a adding: step 1b adding: step 1c

While this process is exactly the same as the standard addition algorithm, it actually provides a conceptual bridge for students because they are able to translate the problem back-and-forth between abstract numbers and the cookie factory universe, helping them reason things through without relying on rules or memory. Grounding their understanding of place value in their intuitive understanding of real-world groups and objects enables students to build more robust schemas for multi-digit arithmetic.

You can use this same approach to multiply multi-digit numbers by one-digit multipliers. Multiplying by multi-digit multipliers will take a little more work.

Generalizing Place Value to Other Units

The same approach that we’ve used here — embedding abstract place value concepts into a concrete cookie factory universe — can be applied to many other contexts. Doing this can help students both transfer and generalize skills and concepts.

We’ve developed similar units using pattern blocks and soda cans. These are intentionally not base ten number systems. Students must discover how to add, subtract, multiply, and divide with pattern block or soda can numbers… and then teach their procedures to others by writing instruction books with algorithms. For pattern blocks, we use semicolons to separate positions, so ( 2 : 1 : 2 ) = 2 hexagons + 1 trapezoid + 2 triangles.

pattern blocks

You can also relate place value to measurement systems by using mixed units, such as ( weeks : days : hours : minutes : seconds ) or ( yards : feet : inches ). And you can introduce decimals if you restrict yourself to pennies, dimes, one-dollar bills, ten-dollar bills, etc. Or let kids make up their own units. One of our beta-testers loves to work in clone trooper units: trooper (ones), squad (tens), company (hundreds), and more!

Place Value from the Ground Up

Before developing Chocolate Chip Cookie Factory: Place Value, Vertical Learning Labs published an interactive multitouch textbook, Place Value from the Ground Up. This textbook also took students through a learning progression for adding, subtracting, multiplying, and dividing multi-digit numbers, but with a slightly different approach. If you are looking for a learning tool with more explicit instruction, we highly recommend it. Check out the videos below to see some of the stages that students pass through.

Blackline Masters

Parallels to Paper-and-Pencil Algorithms

The most efficient processes that a student develops for adding, subtracting, multiplying, and dividing multi-digit cookie orders while playing Chocolate Chip Cookie Factory: Place Value directly parallel the standard paper-and-pencil algorithms that he or she will be using in the classroom.

Addition

	box	stack	cookie
		1
	3	5	8
+	2	7	6
			4

Step 1: Add the cookies together (8 cookies + 6 cookies = 14 cookies)

Step 2: Add 4 cookies to the order and “carry” 1 stack

	box	stack	cookie
	1	1
	3	5	8
+	2	7	6
		3	4

Step 3: Add the 1 stack you carried to the other stacks (1 stack + 5 stacks + 7 stacks = 13 stacks)

Step 4: Add 3 stacks to the order and “carry” 1 box

	box	stack	cookie
	1	1
	3	5	8
+	2	7	6
	6	3	4

Step 5: Add the 1 box you carried to the other boxes (1 box + 3 boxes + 2 boxes = 6 boxes)

Step 6: Add 6 boxes to the order

Multiplication

	box	stack	cookie
		1
	1	6	3
×			4
			2

Step 1: Multiply the cookies (3 cookies × 4 = 12 cookies)

Step 2: Add 2 cookies to the order and “carry” 1 stack

	box	stack	cookie
	2	1
	1	6	3
×			4
		5	2

Step 3: Multiply the stacks (6 stacks × 4 = 24 stacks)

Step 4: Add the 1 stack you carried to the multiplied stacks (1 stack + 24 stacks = 25 stacks)

Step 5: Add 5 stacks to the order and “carry” 2 boxes

	box	stack	cookie
	2	1
	1	6	3
×			4
	6	5	2

Step 6: Multiply the boxes (1 box × 4 = 4 boxes)

Step 7: Add the 2 boxes you carried to the multiplied boxes (2 boxes + 4 boxes = 6 boxes)

Step 8: Add 6 boxes to the order

Subtraction

	box	stack	cookie

	5	1	7
−	2	6	3
			4

Step 1: Deliver 3 cookies; you have 4 cookies left (7 cookies − 3 cookies = 4 cookies)

	box	stack	cookie
	4	11
	5	1	7
−	2	6	3
		5	4

Step 2: Open up 1 box and add the 10 stacks from that box to the other stacks

Step 3: Deliver 6 stacks; you have 5 stacks left (11 stacks − 6 stacks = 5 stacks)

	box	stack	cookie
	4	11
	5	1	7
−	2	6	3
	2	5	4

Step 4: Deliver 2 boxes; you have 2 boxes left (4 boxes − 2 boxes = 2 boxes)

Division

	box	stack	cookie
	1
(÷ 3)	5	5	8
	2

Step 1: Take the 5 boxes and deliver 1 box to each house; you have 2 boxes leftover

box	stack	cookie
1
5	5	8
2	25

Step 2: Open up the 2 leftover boxes and add the 20 stacks from those 2 boxes to the other stacks

	box	stack	cookie
	1	8
	5	5	8
(÷ 3)	2	25
		1

Step 3: Take the 25 stacks and deliver 8 stacks to each house; you have 1 stack leftover

box	stack	cookie
1	8
5	5	8
2	25
	1	18

Step 4: Open up the 1 leftover stack and add the 10 cookies from that stack to the other cookies

	box	stack	cookie
	1	8	6
	5	5	8
	2	25
(÷ 3)		1	18
			0

Step 5: Take the 18 cookies and deliver 6 cookies to each house; you have nothing leftover

Common Core Standards

The skills and concepts developed while filling and delivering orders in Chocolate Chip Cookie Factory: Place Value directly address nine math standards in the Number and Operations in Base Ten (NBT) domain of the Common Core Standards.

1.NBT.2	Understand that the two digits of a two-digit number represent amounts of tens and ones. Understand the following as special cases: 10 can be thought of as a bundle of ten ones - called a “ten.” The numbers from 11 to 19 are composed of a ten and one, two, three, four, five, six, seven, eight, or nine ones. The numbers 10, 20, 30, 40, 50, 60, 70, 80, 90 refer to one, two, three, four, five, six, seven, eight, or nine tens (and 0 ones).
1.NBT.4	Add within 100, including adding a two-digit number and a one-digit number, and adding a two-digit number and a multiple of 10, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method and explain the reasoning used. Understand that in adding two-digit numbers, one adds tens and tens, ones and ones; and sometimes it is necessary to compose a ten.
1.NBT.6	Subtract multiples of 10 in the range 10-90 from multiples of 10 in the range 10-90 (positive or zero differences), using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method and explain the reasoning used.
2.NBT.1	Understand that the three digits of a three-digit number represent amounts of hundreds, tens, and ones; e.g., 706 equals 7 hundreds, 0 tens, and 6 ones. Understand the following as special cases: 100 can be thought of as a bundle of ten tens — called a “hundred.” The numbers 100, 200, 300, 400, 500, 600, 700, 800, 900 refer to one, two, three, four, five, six, seven, eight, or nine hundreds (and 0 tens and 0 ones).
2.NBT.5	Fluently add and subtract within 100 using strategies based on place value, properties of operations, and/or the relationship between addition and subtraction.
2.NBT.7	Add and subtract within 1000, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method. Understand that in adding or subtracting three-digit numbers, one adds or subtracts hundreds and hundreds, tens and tens, ones and ones; and sometimes it is necessary to compose or decompose tens or hundreds.
2.NBT.8	Mentally add 10 or 100 to a given number 100-900, and mentally subtract 10 or 100 from a given number 100-900.
3.NBT.2	Fluently add and subtract within 1000 using strategies and algorithms based on place value, properties of operations, and/or the relationship between addition and subtraction.
3.NBT.3	Multiply one-digit whole numbers by multiples of 10 in the range 10-90 (e.g., 9 × 80, 5 × 60) using strategies based on place value and properties of operations.